Profile picture

Christoph Spiegel

Researcher working on Combinatorics,
Optimization, and Learning

AIS2T @ Zuse Institute
Room 3036 • 14195 Berlin
spiegel@zib.de
Office hours

Wednesdays at ZIB
by e-mail appointment

about me

I am part of Sebastian Pokutta's IOL lab, deputy head of the AIS2T department at ZIB, lead of iol.COMP group, and and a postdoc representative member of the MATH+ Board.


I defended my PhD in additive and probabilistic combinatorics in 2020 at UPC Barcelona under Juanjo Rué and Oriol Serra and currently work on combinatorics, optimization, and learning. In particular, I like using optimization and AI tools for theoretical maths [1] [2] [3], [4], flag algebras [5] [6] [7], [8], and formal proof verification [9]. Click here for a complete list of my publications.


I teach most semesters at either TUB or FUB on topics relating to AI, discrete mathematics, and formal proof verification. My latest course was on formal proof verification with Lean. The course materials can be found on github. Click here for a complete list of courses.


I maintain both the Berlin Math Seminars page and the Formal Proofs from THE BOOK blog.


Also check out my IOL profile.

Publications

proceedings

  1. Zimmer, M., Spiegel, C., and Pokutta, S.
    Compression-aware Training of Neural Networks Using Frank-Wolfe
    Proceedings of the Mathematical Optimization for Machine Learning, 137–168, 2025. [DOI] [arXiv]
  2. Kiem, A., Pokutta, S., and Spiegel, C.
    The Four-color Ramsey Multiplicity of Triangles
    Proceedings of the Discrete Mathematics Days, 2024. [URL] [arXiv] [code]
  3. Zimmer, M., Spiegel, C., and Pokutta, S.
    Sparse Model Soups: A Recipe for Improved Pruning Via Model Averaging
    Proceedings of the International Conference on Learning Representations, 2024. [URL] [arXiv]
  4. Kiem, A., Pokutta, S., and Spiegel, C.
    Categorification of Flag Algebras
    Proceedings of the Discrete Mathematics Days, 2024. [URL]
  5. Mundinger, K., Pokutta, S., Spiegel, C., and Zimmer, M.
    Extending the Continuum of Six-Colorings
    Proceedings of the Discrete Mathematics Days, 2024, April 8. [URL] [arXiv]
  1. Zimmer, M., Spiegel, C., and Pokutta, S.
    Compression-aware Training of Neural Networks Using Frank-Wolfe
    Proceedings of the Mathematical Optimization for Machine Learning, 137–168, 2025. [DOI] [arXiv]
  2. Kiem, A., Pokutta, S., and Spiegel, C.
    The Four-color Ramsey Multiplicity of Triangles
    Proceedings of the Discrete Mathematics Days, 2024. [URL] [arXiv] [code]
  3. Zimmer, M., Spiegel, C., and Pokutta, S.
    Sparse Model Soups: A Recipe for Improved Pruning Via Model Averaging
    Proceedings of the International Conference on Learning Representations, 2024. [URL] [arXiv]
  4. Kiem, A., Pokutta, S., and Spiegel, C.
    Categorification of Flag Algebras
    Proceedings of the Discrete Mathematics Days, 2024. [URL]
  5. Mundinger, K., Pokutta, S., Spiegel, C., and Zimmer, M.
    Extending the Continuum of Six-Colorings
    Proceedings of the Discrete Mathematics Days, 2024, April 8. [URL] [arXiv]
  6. Zimmer, M., Spiegel, C., and Pokutta, S.
    How I Learned to Stop Worrying and Love Retraining
    Proceedings of the International Conference on Learning Representations, 2023. [URL] [arXiv] [code]
  7. Parczyk, O., Pokutta, S., Spiegel, C., and Szabó, T.
    Fully Computer-assisted Proofs in Extremal Combinatorics
    Proceedings of the AAAI Conference on Artificial Intelligence, 2023. [DOI] [URL] [arXiv] [code]
  8. Rué, J. J., and Spiegel, C.
    The Rado Multiplicity Problem in Vector Spaces Over Finite Fields
    Proceedings of the European Conference on Combinatorics, Graph Theory and Applications, 2023, April 1. [DOI] [URL] [arXiv] [code]
  9. Parczyk, O., Pokutta, S., Spiegel, C., and Szabó, T.
    New Ramsey Multiplicity Bounds and Search Heuristics
    Proceedings of the Discrete Mathematics Days, 2022, July 1. [arXiv] [code]
  10. Rué, J. J., and Spiegel, C.
    On a Problem of Sárközy and Sós for Multivariate Linear Forms
    Proceedings of the Discrete Mathematics Days, 2018, February 21. [URL] [arXiv]
  11. Kusch, C., Rué, J. J., Spiegel, C., and Szabó, T.
    Random Strategies Are Nearly Optimal for Generalized Van Der Waerden Games
    Proceedings of the European Conference on Combinatorics, Graph Theory and Applications, 2017, November 20. [URL] [arXiv]

journal

  1. Parczyk, O., Pokutta, S., Spiegel, C., and Szabó, T.
    New Ramsey Multiplicity Bounds and Search Heuristics
    Foundations of Computational Mathematics, 2024. [DOI] [arXiv] [code]
  2. Mundinger, K., Pokutta, S., Spiegel, C., and Zimmer, M.
    Extending the Continuum of Six-Colorings
    Geombinatorics Quarterly, XXXIV, 2024. [URL] [arXiv]
  3. Kamčev, N., and Spiegel, C.
    Another Note on Intervals in the Hales-Jewett Theorem
    Electronic Journal of Combinatorics, 29(1), 2022. [DOI] [URL] [arXiv]
  4. Cao-Labora, G., Rué, J. J., and Spiegel, C.
    An Erdős-Fuchs Theorem for Ordered Representation Functions
    Ramanujan Journal, 56, 183–2091, 2020. [DOI] [URL] [arXiv]
  5. Fabian, D., Rué, J. J., and Spiegel, C.
    On Strong Infinite Sidon and Bₕ Sets and Random Sets of Integers
    Journal of Combinatorial Theory, Series A, 182, 2021. [DOI] [URL] [arXiv]
  1. Parczyk, O., Pokutta, S., Spiegel, C., and Szabó, T.
    New Ramsey Multiplicity Bounds and Search Heuristics
    Foundations of Computational Mathematics, 2024. [DOI] [arXiv] [code]
  2. Mundinger, K., Pokutta, S., Spiegel, C., and Zimmer, M.
    Extending the Continuum of Six-Colorings
    Geombinatorics Quarterly, XXXIV, 2024. [URL] [arXiv]
  3. Kamčev, N., and Spiegel, C.
    Another Note on Intervals in the Hales-Jewett Theorem
    Electronic Journal of Combinatorics, 29(1), 2022. [DOI] [URL] [arXiv]
  4. Cao-Labora, G., Rué, J. J., and Spiegel, C.
    An Erdős-Fuchs Theorem for Ordered Representation Functions
    Ramanujan Journal, 56, 183–2091, 2020. [DOI] [URL] [arXiv]
  5. Fabian, D., Rué, J. J., and Spiegel, C.
    On Strong Infinite Sidon and Bₕ Sets and Random Sets of Integers
    Journal of Combinatorial Theory, Series A, 182, 2021. [DOI] [URL] [arXiv]
  6. Corsten, J., Mond, A., Pokrovskiy, A., Spiegel, C., and Szabó, T.
    On the Odd Cycle Game and Connected Rules
    European Journal of Combinatorics, 89, 2020. [DOI] [URL] [arXiv]
  7. Rué, J. J., and Spiegel, C.
    On a Problem of Sárközy and Sós for Multivariate Linear Forms
    Revista Matemática Iberoamericana, 36(7), 2107–2119, 2020. [DOI] [URL] [arXiv]
  8. Candela, P., Serra, O., and Spiegel, C.
    A Step Beyond Freĭman’s Theorem for Set Addition Modulo a Prime
    Journal De Théorie Des Nombres De Bordeaux, 32(1), 275–289, 2020. [DOI] [URL] [arXiv]
  9. Kusch, C., Rué, J. J., Spiegel, C., and Szabó, T.
    On the Optimality of the Uniform Random Strategy
    Random Structures & Algorithms, 55(2), 371–401, 2018. [DOI] [URL] [arXiv]
  10. Freĭman, G. A., Serra, O., and Spiegel, C.
    Additive Volume of Sets Contained in Few Arithmetic Progressions
    INTEGERS, 19, 2019. [URL] [arXiv]
  11. Rué, J. J., Spiegel, C., and Zumalacárregui, A.
    Threshold Functions and Poisson Convergence for Systems of Equations in Random Sets
    Mathematische Zeitschrift, 288, 333–360, 2017. [DOI] [URL] [arXiv]
  12. Spiegel, C.
    A Note on Sparse Supersaturation and Extremal Results for Linear Homogeneous Systems
    Electronic Journal of Combinatorics, 24(3), 2017. [DOI] [URL] [arXiv]

preprint

  1. Mundinger, K., Zimmer, M., Kiem, A., Spiegel, C., and Pokutta, S.
    Neural Discovery in Mathematics: Do Machines Dream of Colored Planes?
    [arXiv]
  2. Kiem, A., Parczyk, O., and Spiegel, C.
    Forcing Graphs to Be Forcing
    [arXiv]
  3. Parczyk, O., and Spiegel, C.
    An Unsure Note on an Un-Schur Problem
    [arXiv]
  4. Zimmer, M., Andoni, M., Spiegel, C., and Pokutta, S.
    PERP: Rethinking the Prune-Retrain Paradigm in the Era of LLMs
    [arXiv] [code]
  5. Combettes, C., Spiegel, C., and Pokutta, S.
    Projection-free Adaptive Gradients for Large-scale Optimization
    [arXiv] [summary] [code]
  6. Pokutta, S., Spiegel, C., and Zimmer, M.
    Deep Neural Network Training with Frank-Wolfe
    [arXiv] [summary] [code]
  7. Salia, N., Spiegel, C., Tompkins, C., and Zamora, O.
    Independent Chains in Acyclic Posets
    [arXiv]
Talks

Conference and Workshop Talks

October 2024   41st Kolloquium über Kombinatorik (KolKom), Heidelberg [PDF]

September 2024   5th CO@Work Summer school, Berlin [PDF]

July 2024   13th Discrete Mathematics Days (DMD), Alcalá de Henares [PDF]

October 2023   BMS RTA 8 - Practical Course, Berlin [PDF]

August 2023   12th EUROCOMB Conference, Prague [PDF]

October 2024   41st Kolloquium über Kombinatorik (KolKom), Heidelberg [PDF]

September 2024   5th CO@Work Summer school, Berlin [PDF]

July 2024   13th Discrete Mathematics Days (DMD), Alcalá de Henares [PDF]

October 2023   BMS RTA 8 - Practical Course, Berlin [PDF]

August 2023   12th EUROCOMB Conference, Prague [PDF]

August 2023   ICIAM 2023 Minisymposium: Advances in Optimization I, Tokyo [PDF]

August 2023   5th DOxML Conference, Tokyo [PDF]

June 2023   FoCM 2023 Workshop I.3, Paris [PDF]

May 2023   15th CANT Conference, New York [PDF]

March 2023   Workshop on Optimization and ML, Waischenfeld [PDF]

February 2023   37th AAAI Conference, Washington, DC [PDF]

January 2023   Aussois Workshop, Aussois [PDF]

December 2022   Recent Advances in Optimization, Toronto [PDF]

September 2022   6th ZIB-IMI-ISM-NUS-RIKEN-MODAL Workshop, Tokyo / Fukuoka [PDF]

July 2022   12th Discrete Mathematics Days (DMD), Santander [PDF]

June 2019   36th PCC Conference, Oxford [PDF]

June 2019   1st GAPCOMB Workshop, Campelles

June 2018   11th Discrete Mathematics Days (DMD), Sevilla [PDF]

May 2018   10th CANT Conference, New York [PDF]

September 2017   The Music of Numbers Conference, Madrid [PDF]

June 2017   Interactions with Combinatorics [PDF]

March 2017   Joint TAU-FUB Workshop on Graph and Hypergraph Coloring


Seminar Talks

January 2025   Research Seminar AG Discrete Mathematics, Karlsruhe

January 2025   Research Seminar TCS, Heidelberg

December 2024   Research Seminar Combinatorics, Berlin [PDF]

November 2024   Research Seminar Combinatorics, Berlin

February 2023   LIMDA Seminar, Barcelona [PDF]

January 2025   Research Seminar AG Discrete Mathematics, Karlsruhe

January 2025   Research Seminar TCS, Heidelberg

December 2024   Research Seminar Combinatorics, Berlin [PDF]

November 2024   Research Seminar Combinatorics, Berlin

February 2023   LIMDA Seminar, Barcelona [PDF]

April 2022   LIMDA Seminar, Barcelona

November 2019   Research Seminar Combinatorics, Berlin

November 2019   Research Seminar Large Networks and Random Graphs, Ilmenau

May 2019   Seminar on Combinatorics, Games and Optimisation, London

May 2019   Combinatorial Theory Seminar, Oxford

February 2019   Extremal Set Theory Seminar, Budapest

December 2018   Research Seminar Combinatorics, Berlin

March 2018   GRAPHS at IMPA, Rio de Janeiro

December 2017   Research Seminar Combinatorics, Berlin

October 2017   LIMDA Seminar, Barcelona

May 2017   LIMDA Seminar, Barcelona

March 2016   LIMDA Seminar, Barcelona

February 2016   Research Seminar Combinatorics, Berlin

January 2016   Research Seminar Combinatorics, Berlin

December 2015   "What is ...?" seminar

October 2015   Research Seminar Combinatorics, Berlin

Teaching

Winter 2024   Lecturer for Formal Proof Verification at FUB

Summer 2024   Lecturer for Discrete Optimization (ADM II) at TUB

Winter 2023   Lecturer for Introduction to Linear and Combinatorial Optimization (ADM I) at TUB

Summer 2023   Lecturer for Analysis I und Lineare Algebra für Ingenieurwissenschaften at TUB

Autumn 2018   Assistant for Discrete Mathematics and Optimization at UPF

Autumn 2017   Assistant for Discrete Mathematics and Optimization at UPF

Winter 2013   Tutor for Analysis I (Lehramt) at FUB

Summer 2012   Tutor for Analysis II (Lehramt) at FUB

Summer 2011   Tutor for Analysis I (Lehramt) at FUB

Education

June 2020  Ph.D. in Applied Mathematics at Universitat Politècnica de Catalunya   [PDF]

March 2015  Master of Science at Freie Universität Berlin

September 2012  Bachelor of Science at Freie Universität Berlin

Juni 2009  Abitur at Canisius-Kolleg Berlin

Fun Stuff

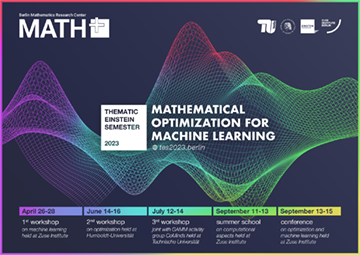
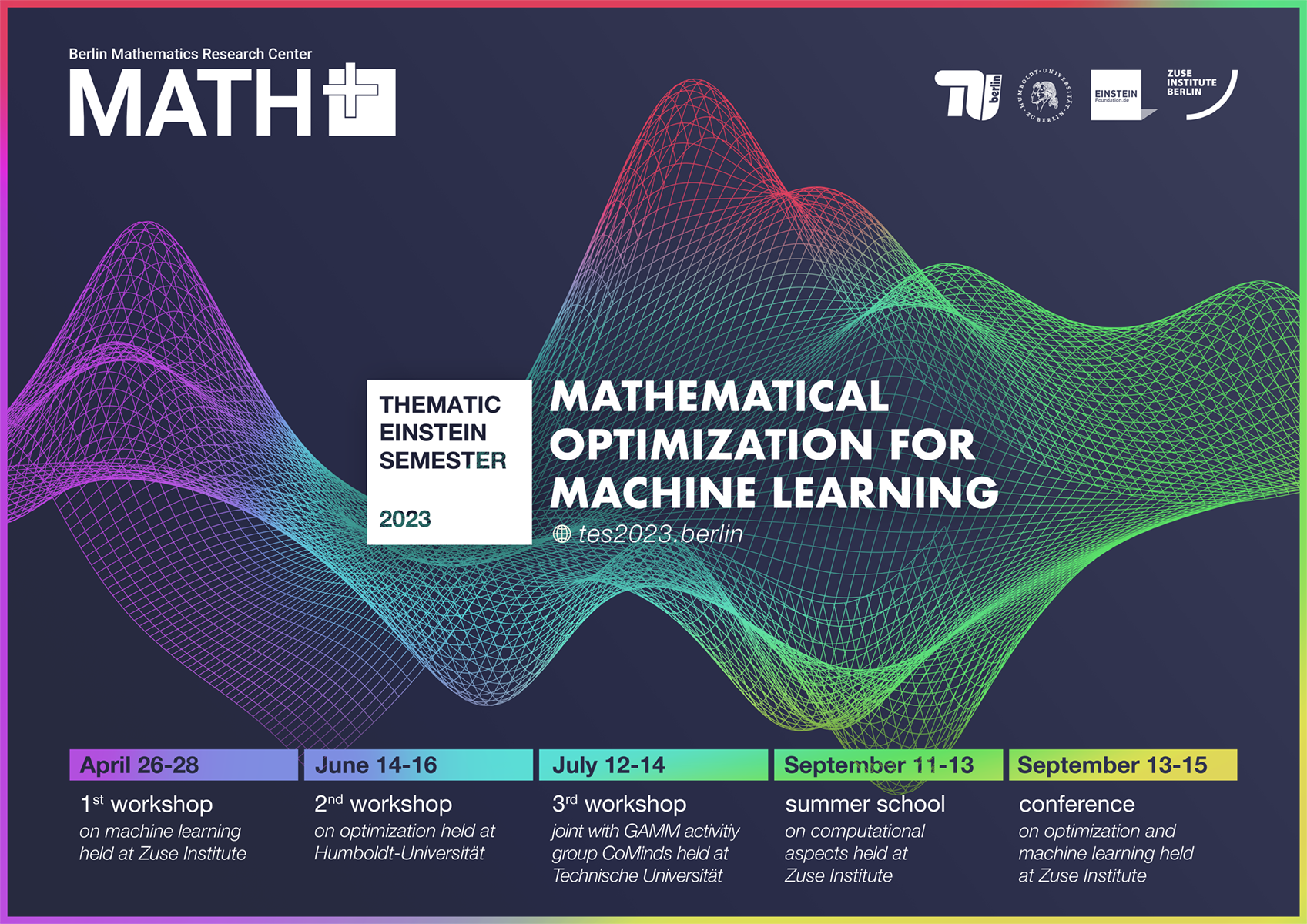
You can find some of my photography on instagram. I also like designing the occasional conference poster:


         






Impressum und Datenschutzerklärung

Impressum

Angaben gemäß § 5 TMG:

Christoph Spiegel
c/o Zuse-Institut Berlin (ZIB), Takustraße 7, 14195 Berlin, Deutschland
E-Mail: spiegel@zib.de, Telefon: +49 30 84185-436

Datenschutzerklärung

1. Verantwortlicher

Der Verantwortliche im Sinne der DSGVO ist die im Impressum genannte Person.

2. Art der Datenerhebung

Bei jedem Aufruf dieser Webseite erfasst der Webserver automatisch folgende Daten: (1) IP-Adresse des anfragenden Rechners, (2) Datum und Uhrzeit des Zugriffs, (3) Name und URL der abgerufenen Datei, (4) Website, von der aus der Zugriff erfolgt, (5) Verwendeter Browser und ggf. das Betriebssystem Ihres Rechners. Diese Daten werden nur zur technischen Bereitstellung der Website benötigt und nach spätestens 7 Tagen gelöscht.

3. Hosting

Der Hoster STRATO AG erhebt in sog. Logfiles folgende Daten, die Ihr Browser übermittelt: IP-Adresse, die Adresse der vorher besuchten Website (Referer Anfrage-Header), Datum und Uhrzeit der Anfrage, Zeitzonendifferenz zur Greenwich Mean Time, Inhalt der Anforderung, HTTP-Statuscode, übertragene Datenmenge, Website, von der die Anforderung kommt und Informationen zu Browser und Betriebssystem. Das ist erforderlich, um diese Website anzuzeigen und die Stabilität und Sicherheit zu gewährleisten. Dies entspricht unserem berechtigten Interesse im Sinne des Art. 6 Abs. 1 S. 1 lit. f DSGVO.

4. Ihre Rechte

Sie haben gegenüber uns folgende Rechte hinsichtlich der Sie betreffenden personenbezogenen Daten: (1) Recht auf Auskunft, (2) Recht auf Berichtigung oder Löschung, (3) Recht auf Einschränkung der Verarbeitung, (4) Recht auf Widerspruch gegen die Verarbeitung, (5) Recht auf Datenübertragbarkeit

Sie haben außerdem das Recht, sich bei einer Datenschutz-Aufsichtsbehörde über die Verarbeitung Ihrer personenbezogenen Daten zu beschweren.